For All In Set Builder Notation
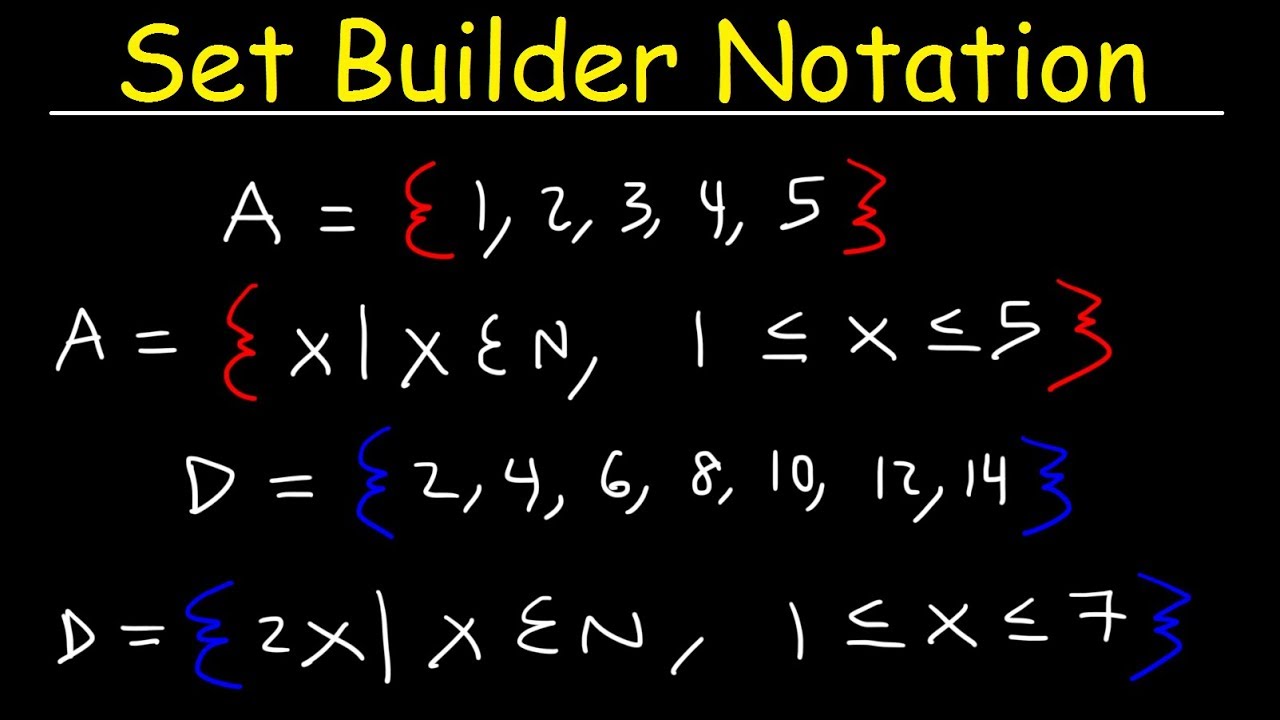
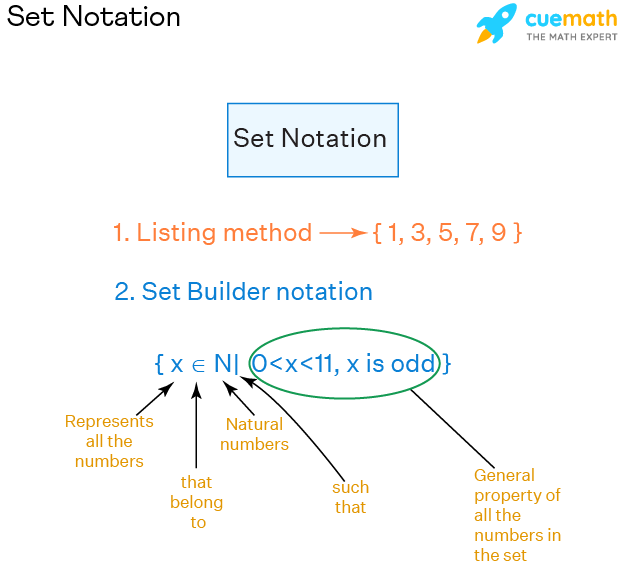
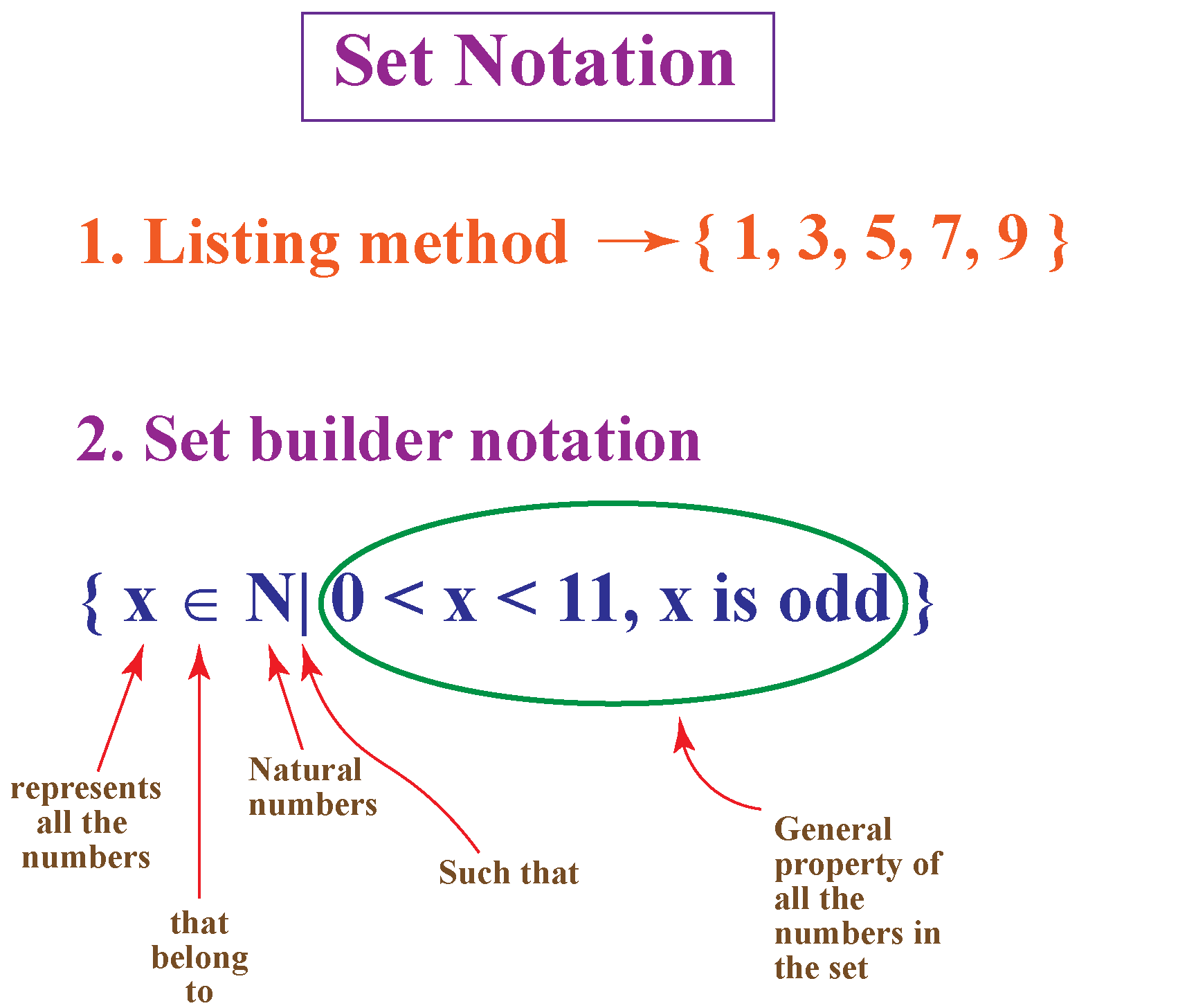
For All In Set Builder Notation - It is particularly useful when dealing with. {a | a ≤ 3} which is. It may be denoted a c or a′. Where (properties of x) is replaced by conditions that fully describe the elements of the set. More generally, we can form a subset of set a. For example, to refer to the set a a of all real numbers from 0 to 5, it is. [1] specifying sets by member properties is. For example, the set { 1, 2, 3, 4, 5, 6, 7, 8, 9 } list the elements. Both a a and b b are defined to be sets of natural numbers, so they can't be equal to s s, which contains fractions. The complement of a is the set of all elements (of u) that do not belong to a. For example, the set { 1, 2, 3, 4, 5, 6, 7, 8, 9 } list the elements. It’s often used in mathematics to define sets in a concise way. It is particularly useful when dealing with. Based on common conventions for set builder notation, i believe the for all or any is implied. More generally, we can form a subset of set a. {a | a ≤ 3} which is. For example, to refer to the set a a of all real numbers from 0 to 5, it is. The complement may also be called the absolute. In mathematics, a set is a. Where (properties of x) is replaced by conditions that fully describe the elements of the set. More generally, we can form a subset of set a. The bar (∣) is used to separate the elements and. Based on common conventions for set builder notation, i believe the for all or any is implied. Start with all real numbers, then limit them to the interval between 2 and 6, inclusive. In mathematics, a set is a. A simple example is written as. An expression, a vertical bar, and a property. Set builder notation is a way to represent sets by using the condition satisfied by every element in the set or a property followed by every element of the set or a limitation on. Normally, one writes ∃x ∈n, n = 2x ∃ x ∈ n,. It may be denoted a c or a′. An important use of predicates is in set builder notation. An expression, a vertical bar, and a property. X ≥ 2 and x ≤ 6 }. We’ll often want to talk about sets that cannot be described very well by listing the elements explicitly or by taking unions,. [1] specifying sets by member properties is. The complement of a is the set of all elements (of u) that do not belong to a. For example, the set { 1, 2, 3, 4, 5, 6, 7, 8, 9 } list the elements. A simple example is written as. The complement may also be called the absolute. For example, the set { 1, 2, 3, 4, 5, 6, 7, 8, 9 } list the elements. The complement of a is the set of all elements (of u) that do not belong to a. It’s often used in mathematics to define sets in a concise way. An expression, a vertical bar, and a property. It is particularly useful. Set builder notation is a way to represent sets by using the condition satisfied by every element in the set or a property followed by every element of the set or a limitation on. In mathematics, a set is a. The complement of a is the set of all elements (of u) that do not belong to a. More generally,. [1] specifying sets by member properties is. An important use of predicates is in set builder notation. A simple example is written as. Set builder notation is a way to represent sets by using the condition satisfied by every element in the set or a property followed by every element of the set or a limitation on. The bar (∣). X ≥ 2 and x ≤ 6 }. Where (properties of x) is replaced by conditions that fully describe the elements of the set. It may be denoted a c or a′. Start with all real numbers, then limit them to the interval between 2 and 6, inclusive. Both a a and b b are defined to be sets of. The bar (∣) is used to separate the elements and. Set builder notation is a way to represent sets by using the condition satisfied by every element in the set or a property followed by every element of the set or a limitation on. The complement of a is the set of all elements (of u) that do not belong. For example, the set { 1, 2, 3, 4, 5, 6, 7, 8, 9 } list the elements. An important use of predicates is in set builder notation. {a | a ≤ 3} which is. Both a a and b b are defined to be sets of natural numbers, so they can't be equal to s s, which contains fractions.. X ≥ 2 and x ≤ 6 }. In mathematics, a set is a. It may be denoted a c or a′. [1] specifying sets by member properties is. It is particularly useful when dealing with. Where (properties of x) is replaced by conditions that fully describe the elements of the set. Normally, one writes ∃x ∈n, n = 2x ∃ x ∈ n, n = 2 x, there. Start with all real numbers, then limit them to the interval between 2 and 6, inclusive. Set builder notation is a way to represent sets by using the condition satisfied by every element in the set or a property followed by every element of the set or a limitation on. An expression, a vertical bar, and a property. {a | a ≤ 3} which is. A simple example is written as. For example, the set { 1, 2, 3, 4, 5, 6, 7, 8, 9 } list the elements. More generally, we can form a subset of set a. The complement of a is the set of all elements (of u) that do not belong to a. It’s often used in mathematics to define sets in a concise way.Set Builder Notation and Roster Method YouTube
Set Builder Notation Cuemath
ShowMe Setbuilder notation
PPT SetBuilder Notation PowerPoint Presentation, free download ID
Set Builder Notation Cuemath
Set Builder Notation Definition, Examples Set Builder Form
Set Builder Notation YouTube
Set builder notation Explanation and Examples
Set Builder Notation Cuemath
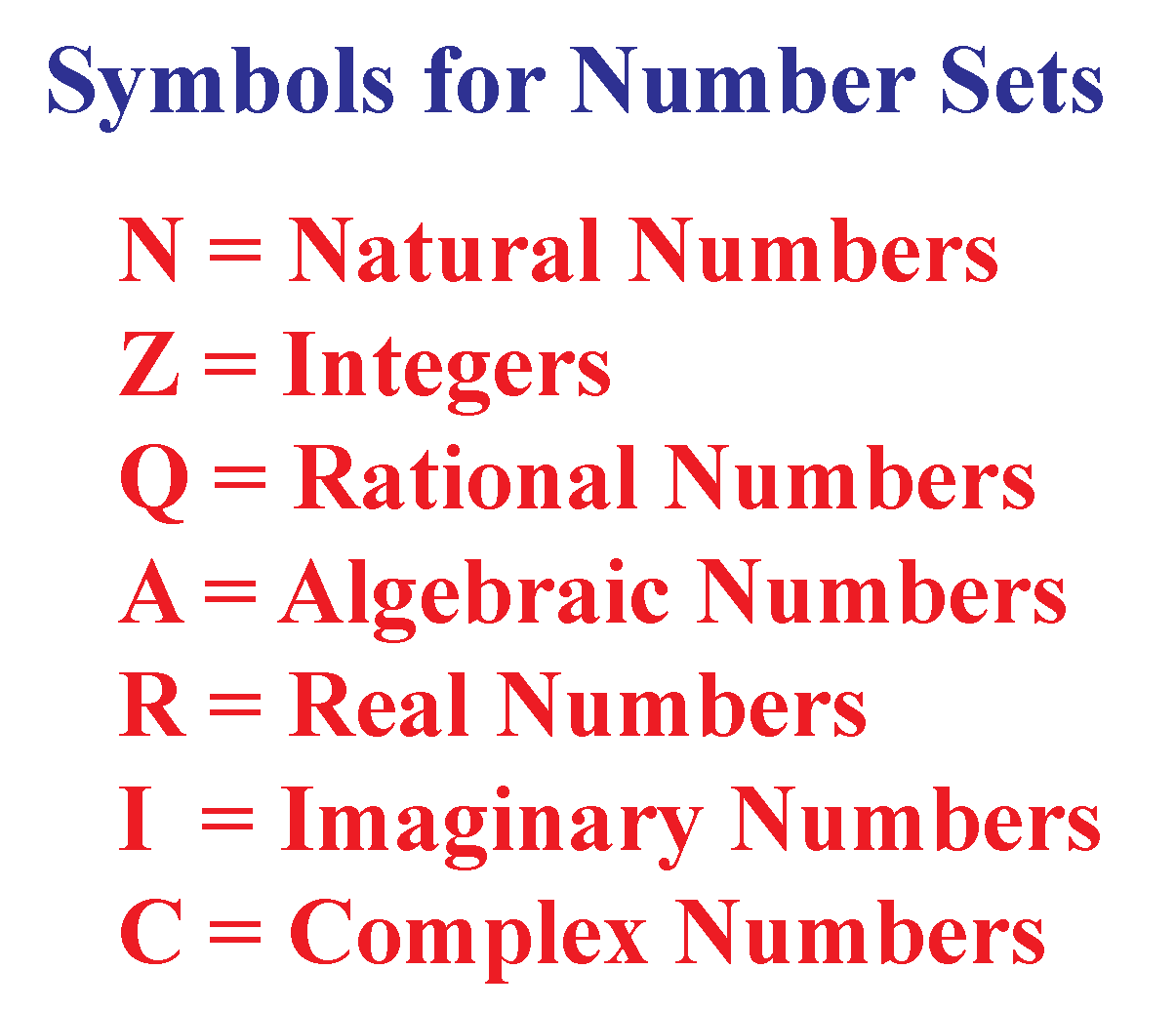
SetBuilder Notation for Algebraic Numbers
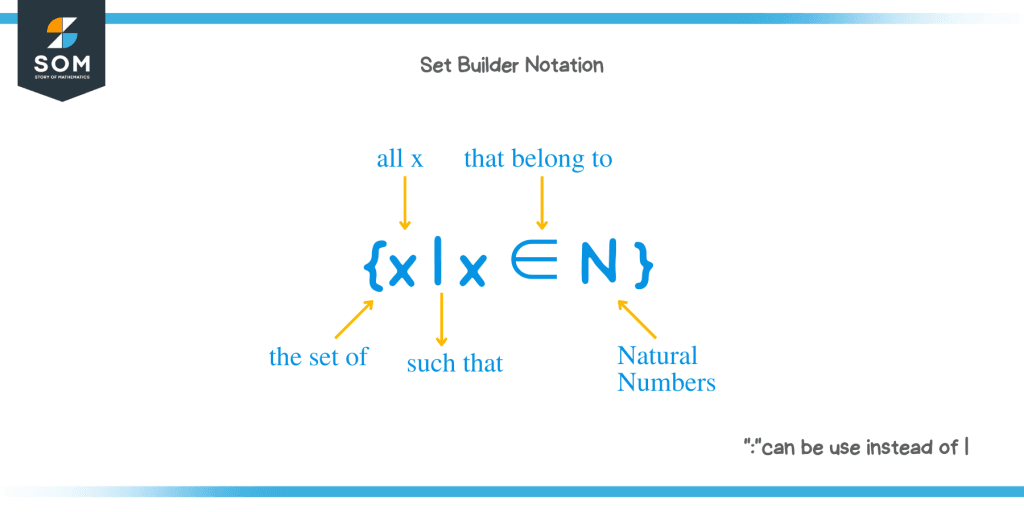
The Bar (∣) Is Used To Separate The Elements And.
Based On Common Conventions For Set Builder Notation, I Believe The For All Or Any Is Implied.
The Complement May Also Be Called The Absolute.
An Important Use Of Predicates Is In Set Builder Notation.
Related Post: