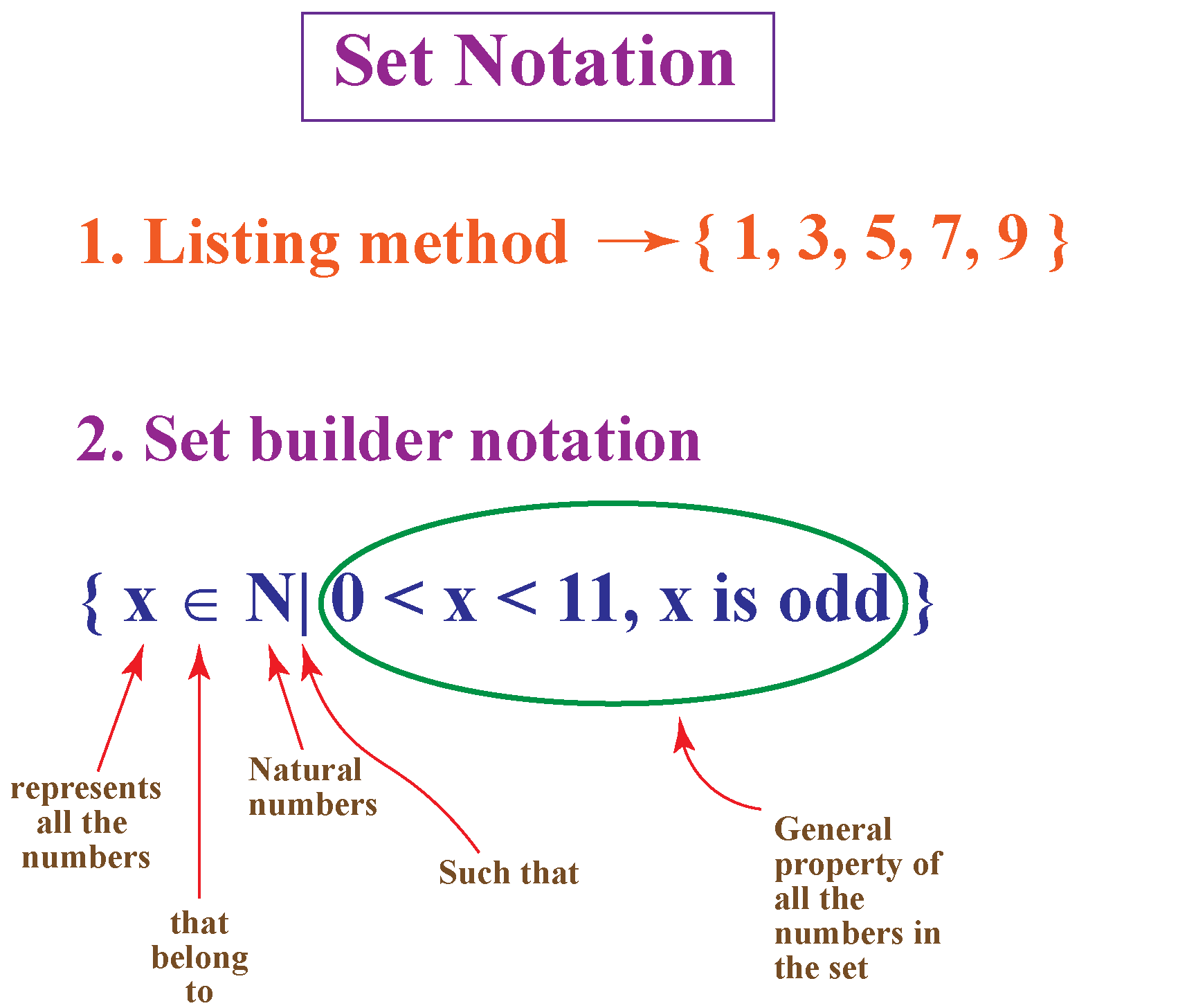
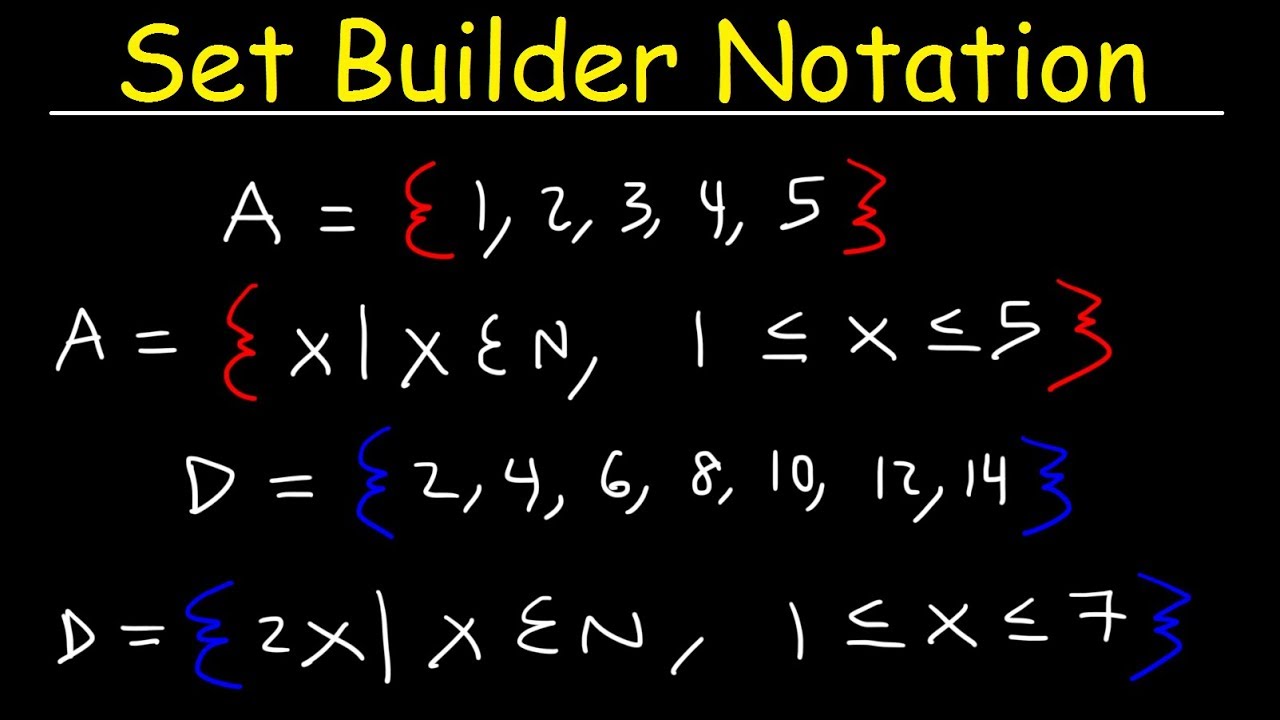
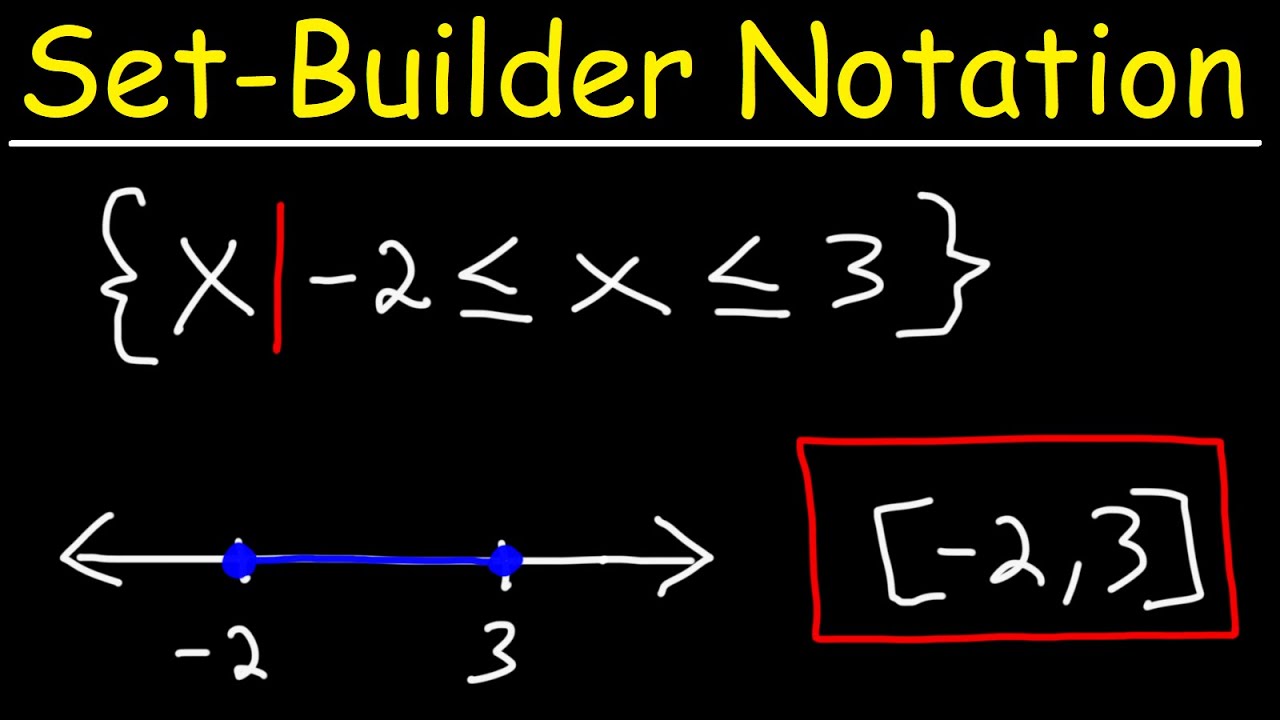
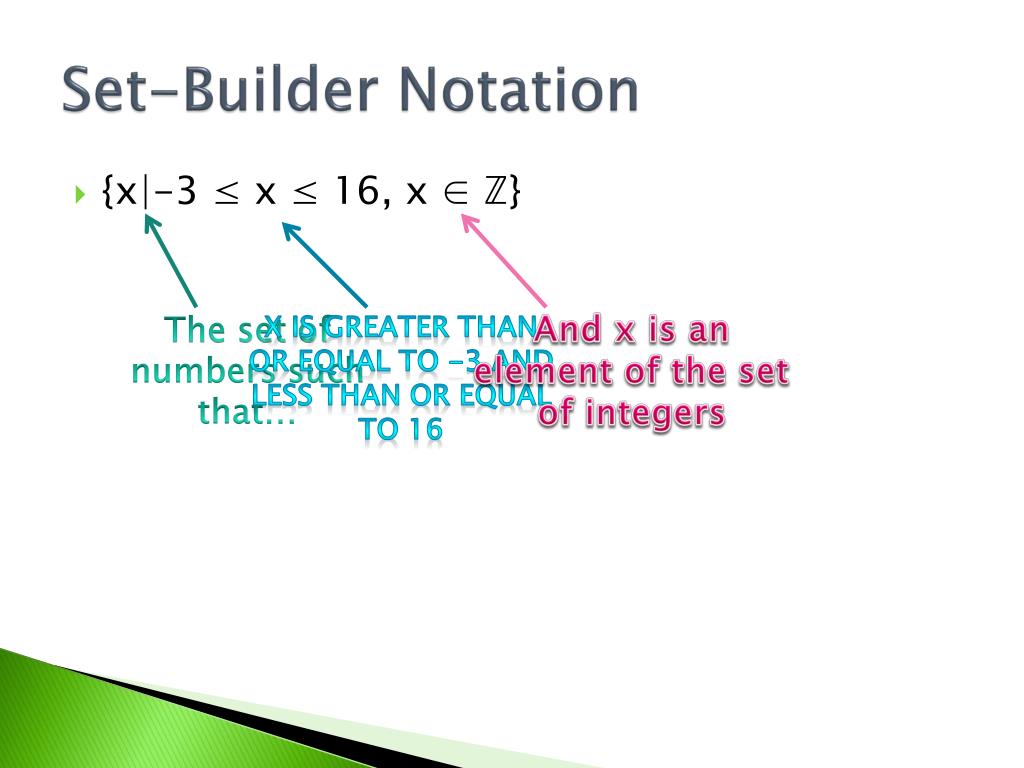
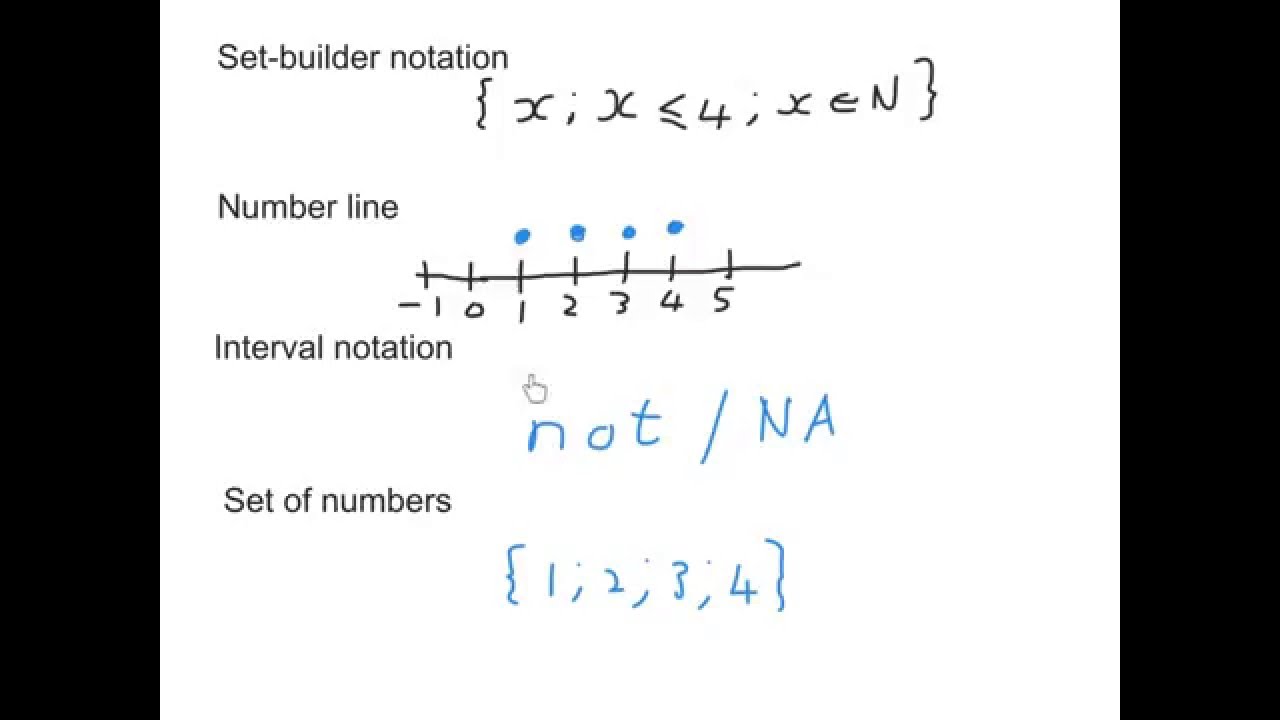
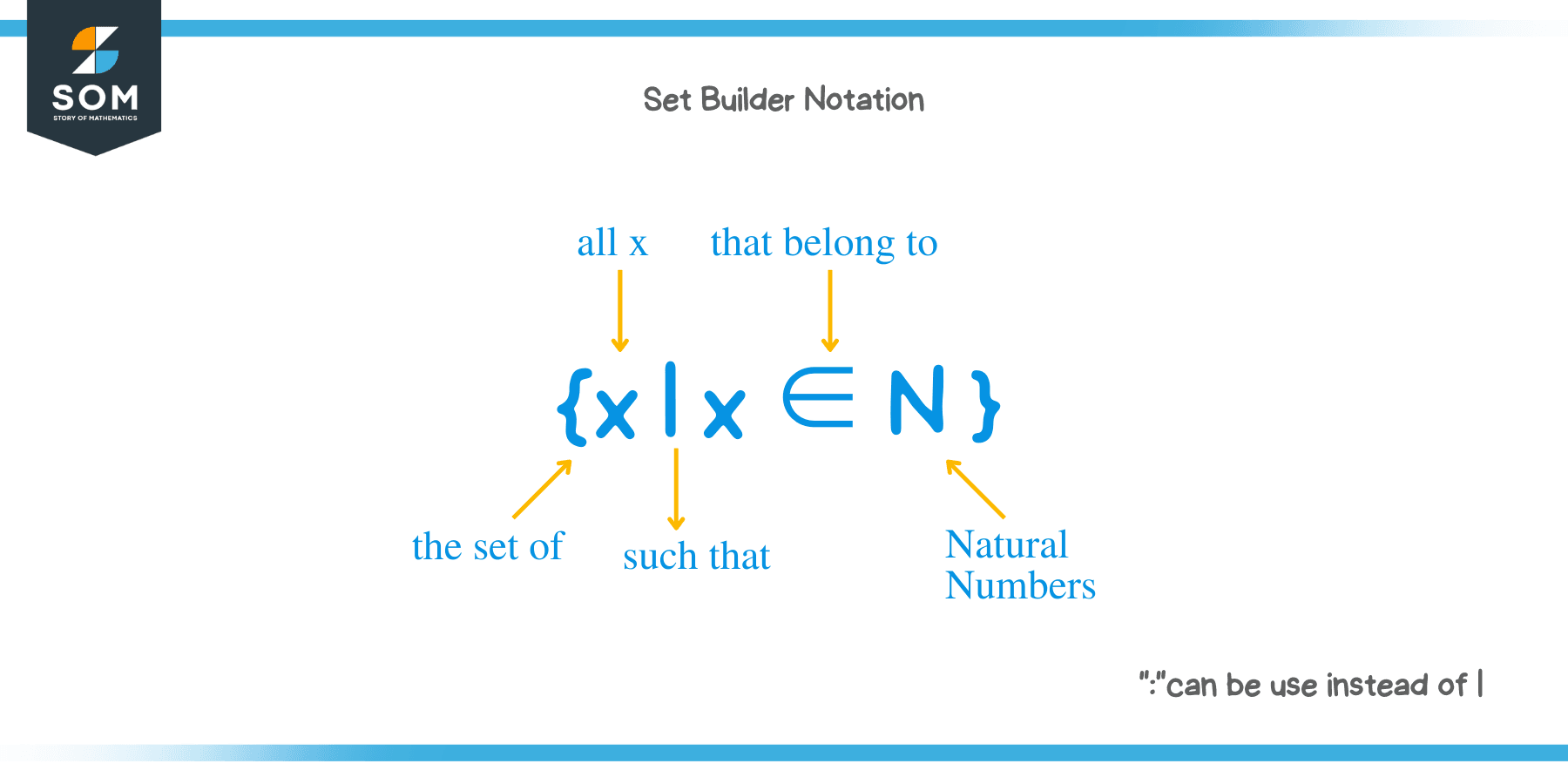
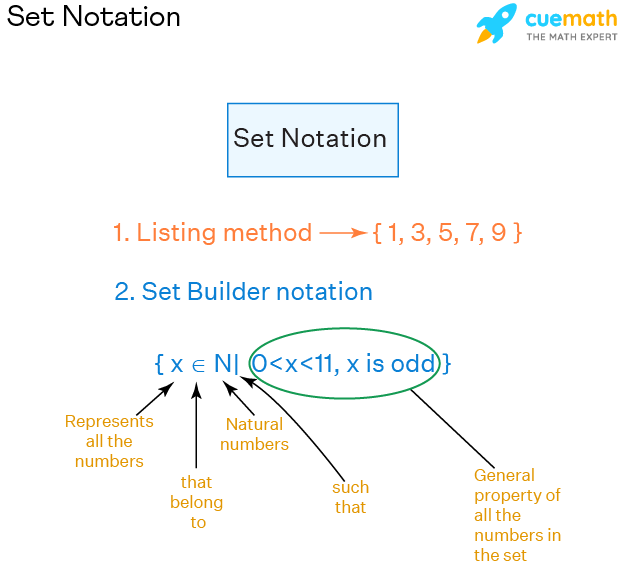
How To Write A Set Builder Notation

How To Write A Set Builder Notation - We’ll often want to talk about sets that cannot be described very well by listing the elements explicitly or by taking. {x | x > 3 } recall that means “a member of”, or simply “in”. The set is written in this form: Set builder notation is a way to represent sets by using the condition satisfied by every element in the set or a property followed by every element of the set or a limitation on. More generally, we can form a subset of set a. In mathematics, set builder notation is a mathematical notation of describing a set by listing its elements or demonstrating its properties that its members must satisfy. It's often useful to define a set in terms of the properties its elements are supposed to have. Denotes the set of all elements in which have the property. For example, look at x below: The same set could be.

It takes the form\(\{x|\text{ statement about x}\}\) which is read as, “the set of all x. An expression, a vertical bar, and a property. {x | x > 3 } recall that means “a member of”, or simply “in”. This is called set builder notation. Also, refer to solved examples on the. It's often useful to define a set in terms of the properties its elements are supposed to have. The set is written in this form: In mathematics, set builder notation is a mathematical notation of describing a set by listing its elements or demonstrating its properties that its members must satisfy. It’s often used in mathematics to define sets in a concise way. Is the special symbol for.

Set Builder Notation Cuemath

More generally, we can form a subset of set a. For example, look at x below: An important use of predicates is in set builder notation. It's often useful to define a set in terms of the properties its elements are supposed to have. We’ll often want to talk about sets that cannot be described very well by listing the.

Set Builder Notation and Roster Method YouTube

Note that the colon : An important use of predicates is in set builder notation. Where (properties of x) is replaced by conditions. It's often useful to define a set in terms of the properties its elements are supposed to have. In mathematics, set builder notation is a mathematical notation of describing a set by listing its elements or demonstrating.

Interval Notation to SetBuilder Notation PreCalculus YouTube

Is the special symbol for. [1] specifying sets by member properties is. For example, the set { 1, 2, 3, 4, 5, 6, 7, 8, 9 } list the elements. It takes the form\(\{x|\text{ statement about x}\}\) which is read as, “the set of all x. An expression, a vertical bar, and a property.

How to Write in Set Builder Notation Form Math Dot Com YouTube

Denotes the set of all elements in which have the property. [1] specifying sets by member properties is. The same set could be. May be replaced by a vertical line |. For example, look at x below:

PPT SetBuilder Notation PowerPoint Presentation, free download ID

May be replaced by a vertical line |. This is called set builder notation. Also, refer to solved examples on the. An expression, a vertical bar, and a property. We’ll often want to talk about sets that cannot be described very well by listing the elements explicitly or by taking.

Notation. three ways,Sets,set builder notation,interval notation,number

We’ll often want to talk about sets that cannot be described very well by listing the elements explicitly or by taking. This site includes a written description and a video describing the three ways to name a set: An expression, a vertical bar, and a property. [1] specifying sets by member properties is. It takes the form\(\{x|\text{ statement about x}\}\).

Set builder notation Explanation and Examples

It's often useful to define a set in terms of the properties its elements are supposed to have. For example, look at x below: An important use of predicates is in set builder notation. We’ll often want to talk about sets that cannot be described very well by listing the elements explicitly or by taking. Denotes the set of all.

Set Builder Notation YouTube

This site includes a written description and a video describing the three ways to name a set: More generally, we can form a subset of set a. {x | x > 3 } recall that means “a member of”, or simply “in”. In mathematics, set builder notation is a mathematical notation of describing a set by listing its elements or.

How To Write In Set Builder Notation

An important use of predicates is in set builder notation. Set builder notation is a way to represent sets by using the condition satisfied by every element in the set or a property followed by every element of the set or a limitation on. Denotes the set of all elements in which have the property. We’ll often want to talk.

Set Builder Notation Cuemath

{x | x > 3 } recall that means “a member of”, or simply “in”. It’s often used in mathematics to define sets in a concise way. Where (properties of x) is replaced by conditions. It's often useful to define a set in terms of the properties its elements are supposed to have. In mathematics, set builder notation is a.

It Takes The Form\(\{X|\Text{ Statement About X}\}\) Which Is Read As, “The Set Of All X.

More generally, we can form a subset of set a. For example, look at x below: An important use of predicates is in set builder notation. A notation used to ''build'' sets by classifying numbers according to their properties.

The Same Set Could Be.

An expression, a vertical bar, and a property. [1] specifying sets by member properties is. For example, the set { 1, 2, 3, 4, 5, 6, 7, 8, 9 } list the elements. We’ll often want to talk about sets that cannot be described very well by listing the elements explicitly or by taking.

This Is Called Set Builder Notation.

Set builder notation is a way to represent sets by using the condition satisfied by every element in the set or a property followed by every element of the set or a limitation on. This site includes a written description and a video describing the three ways to name a set: Denotes the set of all elements in which have the property. Is the special symbol for.

{X | X > 3 } Recall That Means “A Member Of”, Or Simply “In”.

In mathematics, set builder notation is a mathematical notation of describing a set by listing its elements or demonstrating its properties that its members must satisfy. Note that the colon : The set is written in this form: It's often useful to define a set in terms of the properties its elements are supposed to have.